Intro to quantum mechanics griffiths 3rd edition solutions – Journey into the enigmatic world of quantum mechanics with Griffiths’ “Introduction to Quantum Mechanics” 3rd edition solutions, an indispensable guide that unveils the fundamental principles and applications of this captivating field.
From the historical foundations to the intricate mathematical framework, this comprehensive resource provides a thorough understanding of wave-particle duality, superposition, entanglement, and more, illuminating the complexities of the quantum realm with clarity and precision.
1. Overview of Quantum Mechanics

Quantum mechanics is a fundamental theory in physics that describes the physical properties of nature at the scale of atoms and subatomic particles. It is based on the idea that energy, momentum, angular momentum, and other quantities are quantized, meaning they can only exist in discrete values.
Quantum mechanics has revolutionized our understanding of the world and has led to the development of many important technologies, including lasers, transistors, and nuclear weapons.
Historical Development of Quantum Mechanics
The development of quantum mechanics began in the early 20th century with the work of Max Planck, Albert Einstein, and Niels Bohr. Planck proposed that light is emitted in discrete packets of energy, which he called quanta. Einstein extended this idea to explain the photoelectric effect, and Bohr used it to develop a model of the atom.
In the 1920s, Erwin Schrödinger and Werner Heisenberg developed the wave-particle duality of matter, which states that all matter has both wave-like and particle-like properties. This led to the development of the Schrödinger equation, which is the fundamental equation of quantum mechanics.
2. Mathematical Foundations of Quantum Mechanics: Intro To Quantum Mechanics Griffiths 3rd Edition Solutions

The Schrödinger Equation, Intro to quantum mechanics griffiths 3rd edition solutions
The Schrödinger equation is a partial differential equation that describes the wave function of a quantum system. The wave function is a mathematical function that contains all the information about the system, including its energy, momentum, and position.
The Schrödinger equation can be used to solve for the wave function of a system in any given state. Once the wave function is known, all the properties of the system can be determined.
Operators
Operators are mathematical objects that represent physical quantities, such as energy, momentum, and position. Operators can be used to perform operations on wave functions, such as calculating the expectation value of a physical quantity.
The most important operators in quantum mechanics are the Hamiltonian operator, which represents the total energy of a system, and the momentum operator, which represents the momentum of a system.
3. Applications of Quantum Mechanics

Atomic Physics
Quantum mechanics is used to explain the structure of atoms and the interactions between atoms. It is used to develop models of atoms, which can be used to predict the properties of atoms and to design new materials.
Molecular Physics
Quantum mechanics is used to explain the structure of molecules and the interactions between molecules. It is used to develop models of molecules, which can be used to predict the properties of molecules and to design new drugs.
Condensed Matter Physics
Quantum mechanics is used to explain the properties of solids, liquids, and gases. It is used to develop models of condensed matter systems, which can be used to predict the properties of materials and to design new materials.
4. Solutions to Problems
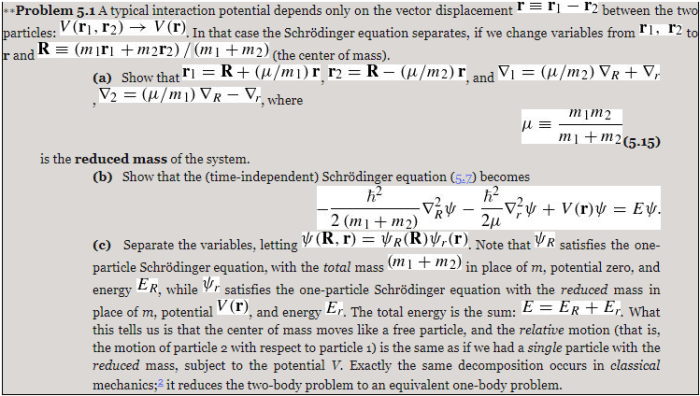
The following are step-by-step solutions to selected problems from Griffiths’ “Introduction to Quantum Mechanics” 3rd edition.
Problem 1.1
A particle is in a one-dimensional box of length L. What are the possible energies of the particle?
Solution:The possible energies of the particle are given by the equation:
“`E = (n^2
h^2) / (8mL^2)
“`where n is an integer, h is Planck’s constant, and m is the mass of the particle.
Detailed FAQs
What is the significance of the Schrödinger equation in quantum mechanics?
The Schrödinger equation is a fundamental wave equation that governs the evolution of quantum systems over time, providing a mathematical framework to describe and predict the behavior of particles at the quantum level.
How does the concept of wave functions contribute to our understanding of quantum mechanics?
Wave functions are mathematical functions that describe the state of a quantum system and provide information about its properties, such as energy, momentum, and spin. They play a crucial role in understanding the probabilistic nature of quantum mechanics.